Rate equation

Editor-In-Chief: C. Michael Gibson, M.S., M.D. [1]

The rate law or rate equation for a chemical reaction is an equation which links the reaction rate with concentrations or pressures of reactants and constant parameters (normally rate coefficients and partial reaction orders). To determine the rate equation for a particular system one combines the reaction rate with a mass balance for the system. For a generic reaction A + B → C the simple rate equation (as opposed to the much more common complicated rate equations) is of the form:

<math>r\; =\; k\left( T \right)\left[ A \right]^{m}\left[ B \right]^{n}</math>

In this equation, <math>[X]</math> expresses the concentration of a given X, usually in mol/litre (molarity). The k(T) is the reaction rate coefficient or rate constant, although it is not really a constant, as it includes everything that affects reaction rate outside concentration such as temperature but also including ionic strength, surface area of the adsorbent or light irradiation.

The exponents n and m are the reaction orders and depend on the reaction mechanism. The stoichiometric coefficients and the reaction orders are very often equal, but only in one step reactions, molecularity (number of molecules or atoms actually colliding), stoichiometry and reaction order must be the same.

Complicated rate equations are not of the form above, and they can be a sum of terms like it or have quantities in the denominator (see further sections)

The rate equation is a differential equation, and it can be integrated in order to obtain an integrated rate equation that links concentrations of reactants or products with time.

If the concentration of one of the reactants remains constant (because it is a catalyst or it is in great excess with respect to the other reactants) its concentration can be included in the rate constant, obtaining a pseudo constant: if B is the reactant whose concentration is constant then <math> r=k[A][B]=k'[A]</math>. The second order rate equation has been reduced to a pseudo first order rate equation. This makes the treatment to obtain an integrated rate equation much easier.

Zero-order reactions

A Zero-order reaction has a rate which is independent of the concentration of the reactant(s). Increasing the concentration of the reacting species will not speed up the rate of the reaction. Zero-order reactions are typically found when a material required for the reaction to proceed, such as a surface or a catalyst, is saturated by the reactants. The rate law for a zero-order reaction is

<math>\ r = k</math>

where r is the reaction rate, and k is the reaction rate coefficient with units of concentration/time. If, and only if, this zero-order reaction 1) occurs in a closed system, 2) there is no net build-up of intermediates and 3) there are no other reactions occurring, it can be shown by solving a Mass balance for the system that:

<math> r = -\frac{d[A]}{dt}=k</math>

If this differential equation is integrated it gives an equation which is often called the integrated zero-order rate law

<math>\ [A]_t = -kt + [A]_0</math>

where <math>\ [A]_t</math> represents the concentration of the chemical of interest at a particular time, and <math>\ [A]_0</math> represents the initial concentration.

A reaction is zero order if concentration data are plotted versus time and the result is a straight line. The slope of this resulting line is the negative of the zero order rate constant k.

The half-life of a reaction describes the time needed for half of the reactant to be depleted (same as the half-life involved in nuclear decay, which is a first-order reaction). For a zero-order reaction the half-life is given by

<math>\ t_ \frac{1}{2} = \frac{[A]_0}{2k}</math>.

Example of a Zero-order reaction

• Reversed Haber process: <math>2NH_3 (g) \rightarrow \; 3H_2 (g) + N_2 (g)</math>

First-order reactions

A first-order reaction depends on the concentration of only one reactant (a unimolecular reaction). Other reactants can be present, but each will be zero-order. The rate law for an elementary reaction that is first order with respect to a reactant A is

<math>\ r = -\frac{d[A]}{dt} = k[A]</math>

k is the first order rate constant, which has units of 1/time.

The integrated first-order rate law is

<math>\ \ln{[A]} = -kt + \ln{[A]_0}</math>

A plot of <math>\ln{[A]}</math> vs. time t gives a straight line with a slope of <math>-k</math>.

The half life of a first-order reaction is independent of the starting concentration and is given by <math>\ t_ \frac{1}{2} = \frac{\ln{(2)}}{k}</math>.

Examples of reactions that are first-order with respect to the reactant:

• <math>\mbox{H}_2 \mbox{O}_2 (l) \rightarrow \; \mbox{H}_2\mbox{O} (l) + \frac{1}{2}\mbox{O}_2 (g)</math>
• <math>\mbox{SO}_2 \mbox{Cl}_2 (l) \rightarrow \; \mbox{SO}_2 (g) + \mbox{Cl}_2 (g)</math>
• <math>2\mbox{N}_2 \mbox{O}_5 (g) \rightarrow \; 4\mbox{NO}_2 (g) + \mbox{O}_2 (g)</math>

Second-order reactions

A second-order reaction depends on the concentrations of one second-order reactant, or two first-order reactants.

For a second order reaction, its reaction rate is given by:

<math>\ r = k[A]^2</math> or <math>\ r = k[A][B]</math>

The integrated second-order rate laws are respectively

<math>\frac{1}{[A]} = kt + \frac{1}{[A]_0} </math> or

<math>\frac{[A]}{[B]} = \frac{[A]_0}{[B]_0} e^{([A]_0 - [B]_0)kt}</math>

[A]₀ and [B]₀ must be different, in order to obtain that integrated equation.

The half-life equation for a second-order reaction dependent on one second-order reactant is <math>\ t_ \frac{1}{2} = \frac{1}{k[A]_0}</math>. For a second-order reaction half-lives progressively double.

Another way to present the above rate laws is to take the log of both sides: <math>\ln{}r = \ln{}k + 2\ln\left[A\right] </math>

Examples of a Second-order reaction

• <math>2\mbox{NO}_2(g) \rightarrow \; 2\mbox{NO}(g) + \mbox{O}_2(g)</math>

Pseudo first order

Measuring a second order reaction rate can be problematic: the concentrations of the two reactants must be followed simultaneously, which is more difficult; or measure one of them and calculate the other as a difference, which is less precise. A common solution for that problem is the pseudo first order approximation

If either [A] or [B] remain constant as the reaction proceeds, then the reaction can be considered pseudo first order because in fact it only depends on the concentration of one reactant. If for example [B] remains constant then:

<math>\ r = k[A][B] = k'[A]</math>

where <math>k'=k[B]_0</math> (k' or k_obs with units s^-1) and we have an expression identical to the first order expression above.

One way to obtain a pseudo first order reaction is to use a large excess of one of the reactants ([B]>>[A] would work for the previous example) so that, as the reaction progresses only a small amount of the reactant is consumed and its concentration can be considered to stay constant. By collecting <math>k'</math> for many reactions with different (but excess) concentrations of [B]; a plot of <math>k'</math> versus [B] gives <math>k</math> (the regular second order rate constant) as the slope.

Summary for reaction orders 0, 1, 2 and n

Reactions with order 3 (called ternary reactions) are very rare, and extremely unlikely to occur. The known ones almost always involve dinitrogen pentoxide (N₂O₅).

	Zero-Order	First-Order	Second-Order	nth-Order
Rate Law	<math>-\frac{d[A]}{dt} = k</math>	<math>-\frac{d[A]}{dt} = k[A]</math>	<math>-\frac{d[A]}{dt} = k[A]^2</math>	<math>-\frac{d[A]}{dt} = k[A]^n</math>
Integrated Rate Law	<math>\ [A] = [A]_0 - kt</math>	<math>\ [A] = [A]_0 e^{-kt}</math>	<math>\frac{1}{[A]} = \frac{1}{[A]_0} + kt</math>	<math>\frac{1}{[A]^{n-1}} = \frac{1}{{[A]_0}^{n-1}} + (n-1)kt</math> [Except first order]
Units of Rate Constant (k)	<math>\frac{M}{s}</math>	<math>\frac{1}{s}</math>	<math>\frac{1}{M \cdot s}</math>	<math>\frac{1}{M^{n-1} \cdot s}</math>
Linear Plot to determine k	<math>[A] \ \mbox{vs.} \ t</math>	<math>\ln ([A]) \ \mbox{vs.} \ t </math>	<math>\frac{1}{[A]} \ \mbox{vs.} \ t</math>	<math>\frac{1}{[A]^{n-1}} \ \mbox{vs.} \ t</math> [Except first order]
Half-life	<math>t_{1/2} = \frac{[A]_0}{2k}</math>	<math>t_{1/2} = \frac{\ln (2)}{k}</math>	<math>t_{1/2} = \frac{1}{[A]_0 k}</math>	<math>t_{1/2} = \frac{2^{n-1}-1}{(n-1)k[A_0]^{n-1}}</math> [Except first order]

Where M stands for concentration (mol · L⁻¹), t for time, and k for for the reaction rate constant.

Equilibrium reactions or opposed reactions

A pair of forward and reverse reactions may define an equilibrium process. For example A and B react into X and Y and vice versa (s, t, u and v are the stoichiometric coefficients):

sA + tB Template:Unicode uX + vY

The reaction rate expression for the above reactions (assuming they each are elementary) can be expressed as:

<math> r = {k_1 [A]^s[B]^t} - {k_2 [X]^u[Y]^v}\,</math>

where: k₁ is the rate coefficient for the reaction which consumes A and B; k₂ is the rate coefficient for the backwards reaction, which consumes X and Y and produces A and B.

The constants k₁ and k₂ are related to the equilibrium coefficient for the reaction (K) by the following relationship (set r=0 in balance):

<math> {k_1 [A]^s[B]^t = k_2 [X]^u[Y]^v}\,</math>

<math> K = \frac{[X]^u[Y]^v}{[A]^s[B]^t} = \frac{k_1}{k_2}</math>

Concentration of A (A₀ = 0.25 mole/l) and B versus time reaching equilibrium k_f = 2 min^-1 and k_r = 1 min^-1

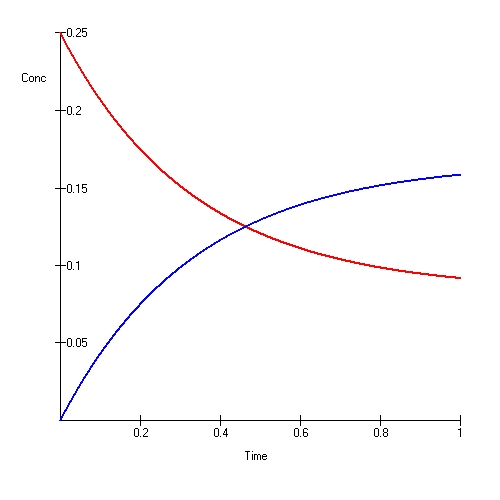
In a simple equilibrium between two species:

<math> A \rightleftharpoons B </math>

the constant K at equilibrium is expressed as:

<math>K \ \stackrel{\mathrm{def}}{=}\ \frac{k_{f}}{k_{b}} = \frac{\left[B\right]_e} {\left[A\right]_e}</math>

When the concentration of A at equilibrium is that of the concentration at time 0 minus the conversion in moles

<math>\ [A]_e = [A]_0 - x </math>

with x equal to the concentration of B at equilibrium

<math>\ [B]_e = x </math>

then it follows that

<math>\ [B]_e = x = \frac{k_{f}}{k_f+k_b}[A]_0 </math>

and

<math>\ [A]_e = [A]_0 - x = \frac{k_{b}}{k_f+k_b}[A]_0 </math>

The reaction rate becomes:

<math>\ \frac{dx}{dt} = \frac{k_f[A]_0}{x_e} (x_e - x) </math>

which results in:

<math>\ ln(\frac{[A]_0 - [A]_e}{[A_t]-[A]_e}) = (k_f + k_b)t </math>

A plot of the negative natural logarithm of the concentration of A in time minus the concentration at equilibrium versus time t gives a straight line with slope k_f + k_b. By measurement of A_e and B_e the values of K and the two reaction rate constants will be known .

When the equilibrium constant is close to unity and the reaction rates very fast for instance in conformational analysis of molecules, other methods are required for the determination of rate constants for instance by complete lineshape analysis in NMR spectroscopy.

Consecutive reactions

If the rate constants for the following reaction are <math>k_1</math> and <math>k_2</math>; <math> A \rightarrow \; B \rightarrow \; C </math>, then the rate equation is:

For reactant A: <math> \frac{d[A]}{dt} = -k_1 [A] </math>

For reactant B: <math> \frac{d[B]}{dt} = k_1 [A] - k_2 [B]</math>

For product C: <math> \frac{d[C]}{dt} = k_2 [B]</math>

These differential equations can be solved analytically and the integrated rate equations (supposing that initial concentrations of every substance except A are zero) are

<math>[A]=[A]_0 e^{-k_1 t}</math>

<math>[B]=[A]_0 \frac{k_1}{k_2 - k_1}\left ( e^{-k_1t}-e^{-k_2t} \right )</math>

<math> [C] = \frac{[A]_0}{k_2-k_1} \left [ k_2 \left ( 1- e^{-k_1t} \right ) - k_1 \left (1- e^{-k_2t} \right ) \right ] \quad = [A]_0 \left (1 + \frac{k_1 e^{-k_2t}-k_2e^{-k_1t}}{k_2-k_1} \right )</math>

The steady state approximation leads to very similar results in an easier way.

Parallel or competitive reactions

When a substance reacts simultaneously to give two different products, a parallel or competitive reaction is said to take place.

• Two first order reactions:

<math> A \rightarrow \; B </math> and <math> A \rightarrow \; C </math>, with constants <math> k_1</math> and <math> k_2</math> and rate equations <math>-\frac{d[A]}{dt}=(k_1+k_2)[A]</math>, <math> \frac{d[B]}{dt}=k_1[A]</math> and <math> \frac{d[C]}{dt}=k_2[A]</math>

The integrated rate equations are then <math>\ [A] = [A]_0 e^{-(k_1+k_2)t}</math>; <math>[B] = \frac{k_1}{k_1+k_2}[A]_0 (1-e^{-(k_1+k_2)t})</math> and <math>[C] = \frac{k_2}{k_1+k_2}[A]_0 (1-e^{-(k_1+k_2)t})</math>.

One important relationship in this case is <math> \frac{[B]}{[C]}=\frac{k_1}{k_2}</math>

• One first order and one second order reaction:

This can be the case when studying a bimolecular reaction and a simultaneous hydrolysis (which can be treated as pseudo order one) takes place: the hydrolysis complicates the study of the reaction kinetics, because some reactant is being "spent" in a parallel reaction. For example A reacts with R to give our product C, but meanwhile the hydrolysis reaction takes away an amount of A to give B, a byproduct: <math> A + H_2O \rightarrow \ B </math> and <math> A + R \rightarrow \ C </math>. The rate equations are: <math> \frac{d[B]}{dt}=k_1[A][H_2O]=k_1'[A]</math> and <math> \frac{d[C]}{dt}=k_2[A][R]</math>. Where <math>k_1'</math> is the pseudo first order constant.

The integrated rate equation for the main product [C] is <math> [C]=[R]_0 \left [ 1-e^{-\frac{k_2}{k_1'}[A]_0(1-e^{-k_1't})} \right ] </math>, which is equivalent to <math> ln \frac{[R]_0}{[R]_0-[C]}=\frac{k_2[A]_0}{k_1'}(1-e^{-k_1't})</math>. Concentration of B is related to that of C through <math> [B]=-\frac{k_1'}{k_2} ln \left ( 1 - \frac{[C]}{[R]_0} \right )</math>

The integrated equations were analytically obtained but during the process it was assumed that <math>[A]_0-[C]\approx \;[A]_0</math> therefeore, previous equation for [C] can only be used for low concentrations of [C] compared to [A]₀

See also

• Reaction rate
• Reaction rate constant
• Reactions on surfaces: rate equations for reactions where, at least one of the reactants adsorbs onto a surface
• Steady state approximation